Grand canonical ensemble

Grand canonical ensemble

• Microcanonical ensemble is a collection of independent assemblies in which energy (E), volume (V), and number of particles (N) remain constant.
• Canonical ensemble is a collection of independent assemblies in which temperature (T), volume (V), and number of particles (N) remain constant.
• It mean a microcanonical ensemble ⟶ Canonical ensemble, if we ignore the condition E = constant.
• Therefore the energy exchange takes place in this ensemble.
• Actually in chemical process, the number of particles N varies and it is very difficult to keep the number of particles constant in various phenomenon like radioactive decay process.
• Thus grand canonical ensemble is an ensemble in which the exchange of energy as well as the number of particles takes place with the heat reservoir.
• The grand canonical ensemble is a collection of essentially independent assemblies having the same temperature T, volume V, and a chemical potential µ.

• Thus grand canonical ensemble is a situation in which we know both the average energy and the average number of particles in assembly, otherwise we don’t know the state of the system.
• The density function in Гspace for grand canonical ensemble = ρ (p, q, N)
• Here p is momenta, q is coordinate and N is number of particles.

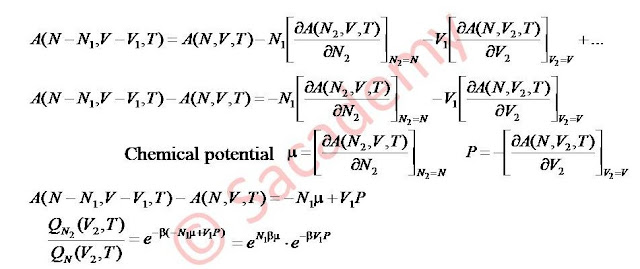
• To find ρ (p, q, N), we consider the canonical ensemble for a system with particles N, volume V and temperature T.
• Here we can not take exactly V = constant, so our focus is on a small sub-volume V₁ of the system.
• Let N₁ particles be in volume V₁, and N₂ particles be in volume V₂
• N₁ + N₂ = N   ⇒   N₂ = N – N₁    and    V₁ + V₂ = V   ⇒   V₂ = V – V₁
• Also we assume that V₂ >> V₁ and N₂ >> N₁
• If H₁ and H₂ be the Hamiltonian of the system then
• The total Hamiltonian of the composite system
• H (p, q, N) = H₁ (p₁, q₁, N₁) + H₂ (p₂, q₂, N₂)

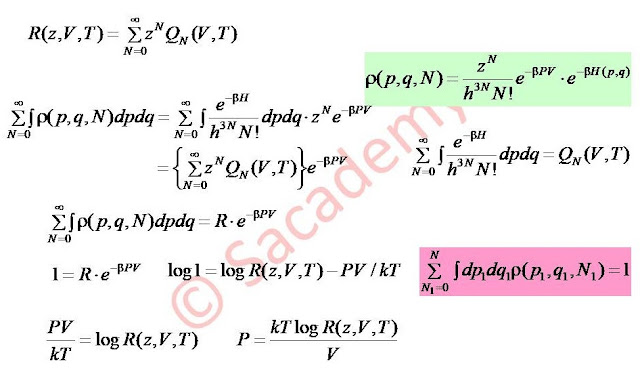
Partition function of the system

• The partition function of the total system

• Here A (N, V, T) is the Helmholtz free energy.
  

• In the last term we consider that the system under consideration to become infinite in size or 0 ≤ N < ∞.
• For thermodynamic function, we define grand partition function

Average number of particle

• By ensemble average, the average number of particles

Internal energy