Microcanonical ensemble

Microcanonical ensemble

• It is a collection of essentially independent assemblies having the same energy E, volume V, and number of system N.
• All the systems are of same type.
• The independent assemblies are separated by rigid, impermeable and well insulated walls such that the values of E, V and N are not affected by the presence of other system.
• We can not actually specify the macroscopic energy of an assembly exactly.

• We consider two neighbouring surfaces with energies E and E + ΔE.
• In macroscopic ensemble every system has N molecules, V volume and energy between E and E + ΔE
• Also the average value of total momentum of the system = 0

• Let Γ(E) = Volume in Γ-space occupied by the microcanonical ensemble.

• Let Σ(E) = Volume in Γ-space enclosed by the energy surface of energy E

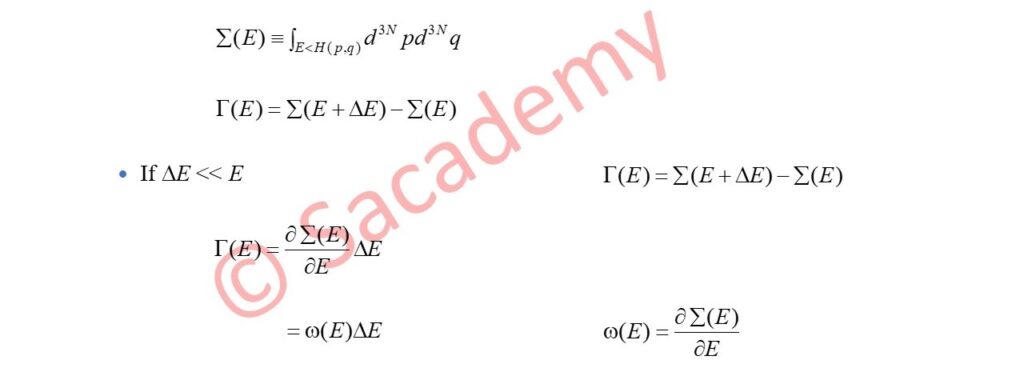
• Here ω(E) = Density of the system at the energy E.
• If S = Entropy of the system, and k = Boltzmann’s constant, then.

          S (E, V) = k log Γ (E)

S is an extensive property

• If a system is composed of two sufficient large sub-systems having entropies S1 and S2, then the entropy of the total system is

          S = S₁ + S₂

• Let a system be composed of two sub-systems having N₁ and N₂ particles in the subsystems and V₁ and V₂ be the volumes corresponding to these subsystems respectively.
• If H₁ (p₁, q₁) and H₂ (p₂, q₂) are the Hamiltonian of the two subsystems then the total Hamiltonian of the composite system is

          H (p, q) = H₁ (p₁, q₁) + H₂ (p₂, q₂)

• Let the two subsystems be isolated from each other
• The energy of first subsystem lie between E₁ and E₁ + ΔE and
• The energy of second subsystem lie between E₂ and E₂ + ΔE.

∴        The entropies of the two subsystems are

          S₁ (E₁, V₁) = k log Γ₁ (E₁)  and  S₂ (E₂, V₂) = k log Γ₂ (E₂)

• Here Γ₁ (E₁) and Γ₂ (E₂) are the volumes occupied by the two ensembles in their Γ space.

• Let the composite system be made up of two subsystems and the microcanonical ensemble of it lie between energy range E and E + 2ΔE
• Also ΔE << E

• In the microcanonical ensemble of composite system

(i) N₁ particles whose momenta and coordinate are p₁, q₁ contained in the volume V₁

(ii) N₂ particles whose momenta and coordinate are p₂, q₂ contained in the volume V₂

(iii) The energies E₁ and E₂ of the subsystem satisfy the condition

          E < (E₁ + E₂) < E + 2 ΔE

∴     The total volume of the region of Γ-space corresponding to (i) and (ii) and energy lying between (E₁ + E₂) and (E₁ + E₂) + 2ΔE is

          Γ₁ (E₁) Γ₂ (E₂)

• For total volume of the ensemble Γ (E), we sum of Γ₁ (E₁) and Γ₂ (E₂) over E₁ and E₂.
• For simplicity we take lower bound  for both systems to be 0 and divide each of energy spectra into internal of size ΔE, between 0 and E.
• Therefore there are E/ΔE intervals in each spectra.

• Where E_i = energy lying in the centre of each energy internal.

• If the number of particles of composite system N = N₁ + N₂
• Volume of composite system V = V₁ + V₂
• Entropy of composite system is

          Since ΔE = constant (independent of N)

∴        log (E/ΔE) may be neglected

Conclusion

• Above result proves the extensive property of the entropy.
• The energies of subsystems have the definite values E₁ (bar) and E₂ (bar) respectively.
• E1 and E2 maximize the function Γ₁ (E₁) Γ₂ (E₂),  when E₁ + E₂  < E

∴      δ { Γ₁ (E₁) Γ₂ (E₂) } = 0           ;    when  δE₁ + δE₂ = 0

or    δ log Γ₁ (E₁) = – δ log Γ₂ (E₂)  ;    when  δE₁ = –  δE₂

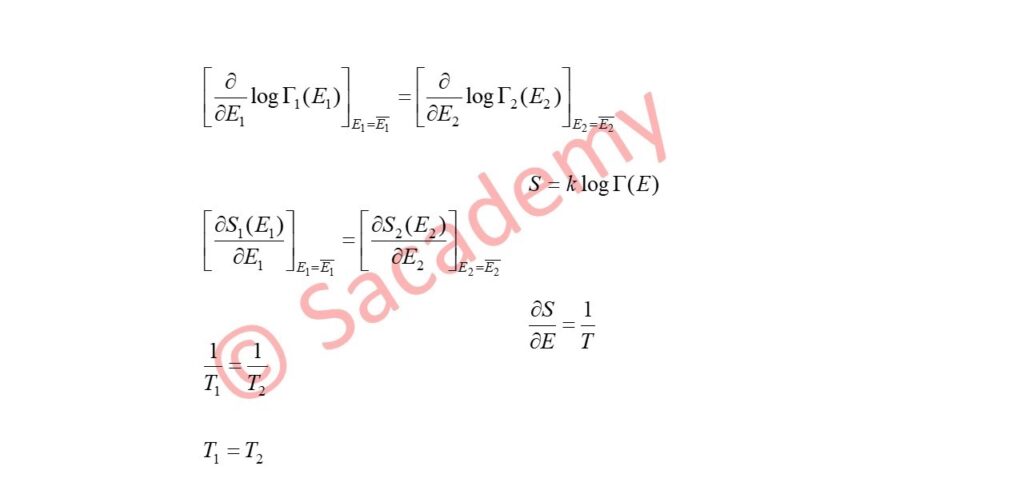
• Thus E₁ (bar) and E₂ (bar) are such that the two subsystems have the same temperature.
• Thus T is a parameter associated with the condition of equilibrium and it is also related with energy.
• Thus the proof of extensive property of entropy shows that temperature of an isolated system is the parameter representing the equilibrium between one part of the system and another.
• This equation shows that S = k log Γ (E)  ;  S = k log ω (E)  and  S = k log Σ (E) are equivalent up to additive constant terms of order log N {O (log N)} or smaller.

S satisfies the properties of the entropies required by the second law of thermodynamics

• In thermodynamics, the entropy (S) is defined only for equilibrium state.
• According to 2nd law of T.D., if an isolated system undergoes a change of thermodynamic state such that the initial and final state are equilibrium state, the entropy of the final state is not smaller than that of the initial state.

∴      S_f ≥  S_i

• Let N, V and E are independent macroscopic parameters of a system.
• If system is isolated then N and E are constant.
• Therefore only V can change.
• But V can not decrease, without compressing the system or disturbing its isolation.
• So V can only increase.
• Therefore the second law of TD states that S is a non-decreasing function of V.
• Hence S defined by any of the equation S = k log Γ (E)  ;  S = k log ω (E)  and  S = k log Σ (E), represents that S of a system is a function of volume V and internal energy E.
• This represents the connection between the microcanonical ensemble and thermodynamics. as