Compound pendulum | Oscillations and Waves

Sacademy May 7, 2023 2 min read

Compound pendulum (Physical pendulum)

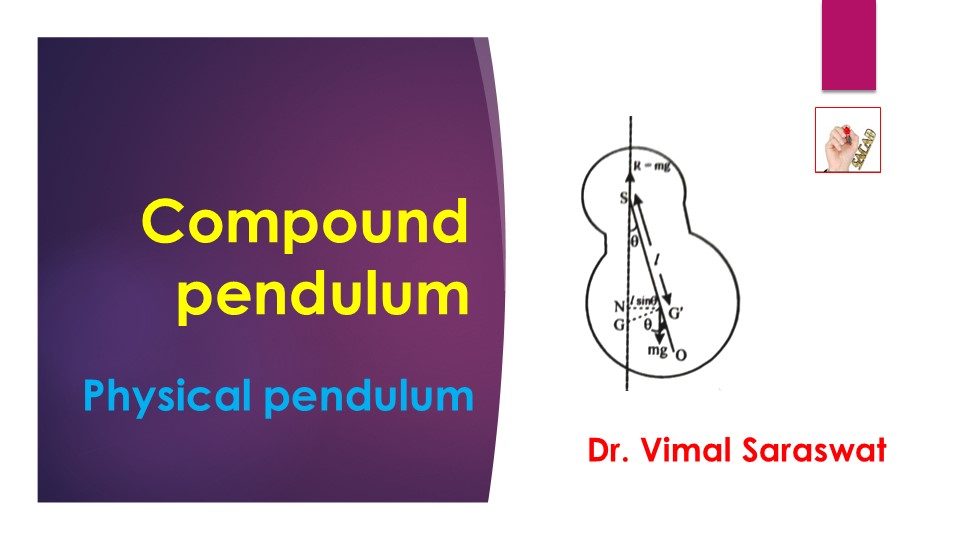
A rigid body capable of oscillating in a vertical plane about a horizontal axis passing through the body, but not through the centre of gravity is compound pendulum.
Let the mass of compound pendulum, which is in the form of a rigid body be m.
Let this pendulum be suspended about a horizontal axis passing through S (point of suspension).
G = centre of gravity, and SG = l
In equilibrium, G lies vertically below S.
Now the body is slightly displaced through an angle θ so that the G is shifted to G’.
When body is slightly displaced, then a restoring couple acts on the body due to its weight, which tries to move the body towards the equilibrium position.

Restoring couple τ = – mg× G’N
or τ = – mgl sin θ
If I is moment of inertia of body about the horizontal axis passing through S and α is the angular acceleration, then
Deflecting couple

$\; \; \; \; \; \tau _{1}=I\alpha =I\frac{d^{2}\theta }{dt^{2}}$

$or \;\;\;\;\; I\frac{d^{2}\theta }{dt^{2}}=-mglsin\theta \;\;\;\;\;\left ( \because\tau =-mglsin\theta \right )$

$or \;\;\;\;\; \frac{d^{2}\theta }{dt^{2}}+\frac{mgl}{I}sin\theta=0$

$or \;\;\;\;\; \frac{d^{2}\theta }{dt^{2}}+\omega_{0}^{2}sin\theta=0 \;\;\;\;\;\left ( \because \omega _{0}=\sqrt{\frac{mgl}{I}} \right )$

$\therefore \;\;\;\;\; \frac{d^{2}\theta }{dt^{2}}+\omega_{0}^{2}\theta=0$

Time period

T = 2π/ω₀
Since ω₀ = √(mgl/I)
Therefore T = 2π √ (I/mgl)
If I₀ be the moment of inertia of the body about an axis passing through the centre of gravity, then from parallel axis theorem, I = I₀ + ml²
If k = radius of gyration, about an axis passing through the centre of gravity, then

$I = mk^{2}+ml^{2}=m(k^{2}+l^{2})$

$\because \;\;\;\;\;\ T =2\pi \sqrt{\frac{I}{mgl}}$

$\therefore \;\;\;\;\;\ T =2\pi \sqrt{\frac{m(k^{2}+l^{2})}{mgl}}=2\pi \sqrt{\frac{k^{2}+l^{2}}{gl}}$

$or \;\;\;\;\;\ T =2\pi \sqrt{\frac{l+k^{2}/l}{g}}$

The time period of simple pendulum with effective length L is T = 2π √ (L/g)
Thus time period of simple pendulum and compound pendulum is same with effective length L = l + k²/ l
So L = l + k²/ l is called the length of equivalent simple pendulum.
If we extend SG’ to O such that SO = l + k²/ l
This time O is called the centre of oscillation.
Here G’O = k²/ l = l‘ (say)
So L = l + l‘
Hence T = 2π √ {(l + l‘)/g}
If we interchange the point of suspension and point of oscillation, then new time period is

$\;\;\;\;\;\ T' =2\pi \sqrt{\frac{l'+k^{2}/l'}{g}}$
$Here \;\;\;\;\;\ \frac{k^{2}}{l}=l'\;\;\;\;\;\;\frac{k^{2}}{l'}=l$

$\therefore \;\;\;\;\;\ T' =2\pi \sqrt{\frac{l'+l}{g}}$

To know more about Compound pendulum click here.