Chromatic aberration and its reduction

Aberration

• The spherical surfaces and lenses are used to create the images of given objects.
• If we find the position, size and forms of images by using simple equations, then there will be several defects or aberration in images.
• The defects in images formed by a lens or combination of lenses are known as aberration.

Chromatic aberration or Colour defect

• When white light incident on a prism, then after refraction form the prism it splits into seven colours.
• In the same way if white light incident on a lens and after refraction from the lens, we get the images of different colours, then such defect of lens is known as chromatic aberration or colour defect.
• Because a lens can be assumed as a combination of several prisms, and their angle of refraction decreases as we go from centre to the corner.

Reason

• The refractive index of lens material is different for different colours or the light of different wavelengths.
• The light of different wavelengths focuses at different and forms the image at different positions.
• From Cauchy relation  µ = A + B/λ²

∵     λ_R > λ_{V     ⇒     µR < µV}

∵     f ∝ 1/µ     _{⇒     fR > fV}

• Since the focal length of red is highest, so the deviation of red is minimum and the deviation of violet is maximum.

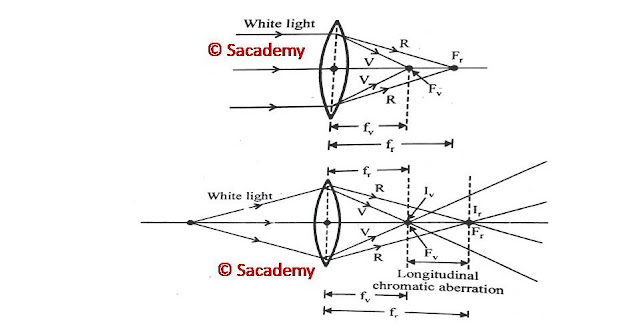
• (f_r ー f_v) measures the axial or longitudinal chromatic aberration.

Longitudinal chromatic aberration

• The difference of focal length of lens for red and violet colour provides the measure of axial or longitudinal chromatic aberration.
• Since focal length of lens

• But dispersive power of lens material is ω , so

• If f_y = focal length of mean colour, then f_y² = f_v f_r
• f_r − f_v = ωf_y
• Thus longitudinal chromatic aberration is the product of dispersive power and focal length of ray of mean colour.

Transverse chromatic aberration

• If a white light object is placed normal to the axis of a lens then it is imaged normal to the axis.
• Since the refractive index of lens is different for different colours and hence the image formed normal to the axis will be of different colours and of different sizes.
• If I = size of image, O = size of object, u = distance of object from the optical centre of lens and v = distance of image from the optical centre of lens, then
• Magnification m = I/O = v/u

• AB = White object
• A_vB_v = Violet colour image of given white object
• A_rB_r = Red colour image of given white object
• A_rB_r ー A_vB_v = Measure of transverse chromatic aberration
• M_r ー M_v = Transverse chromatic aberration in terms of magnification

• If the size of red image is greater than the size of violet image (M_r > M_v), then the transverse chromatic aberration is said to be positive (aberration due to convex lens).
• If M_r < M_v, then the transverse chromatic aberration is said to be negative (aberration due to concave lens).

Analytically

• The rate of change of size of image with respect to the wavelength is chromatic aberration.
• If x = axial distance, and y = transverse distance, then
• Longitudinal chromatic aberration = dx/dλ
• Transverse chromatic aberration = dy/dλ

• The process of minimizing chromatic aberration is known as achromatism.
• There are two methods for getting achromatism
• By an achromatic doublet in which two lenses convex (crown glass) and concave (flint glass) are placed in contact.
• By placing two convex lenses of same material at a suitable distance.

Achromatic combination of two lenses in contact

• The combination of two or more lenses are arranged in such a way that the image obtained from it is free from chromatic aberration is achromatic lens.
• For convex lens f_v < f_r and for concave lens f_v > f_r
• Chromatic aberration of convex lens is positive.
• Chromatic aberration of concave lens is negative.
• To obtain the image free from chromatic aberration, the two lenses (one is convex and another is concave) are grouped together. Such arrangement is known as achromatic doublet.
• For achromatic doublet, we use a crown glass of high power (or low focal length) and a flint glass of low power (or large focal length).
• The focal length of two lenses are so adjusted that the focus of violet coincides with the focus of red.

• Since focal length of thin lens is

• Let f₁ and f₂ be the focal length of crown and flint glass respectively, then the focal length of combination will be

• For achromatism F = constant ⇒ dF = 0

• Since ω₁ / ω₂ = ー f₁ / f₂ 
• And ω₁ and ω₂ both are positive, so f₁ and f₂ must be of opposite sign. It means if one lens is convex, the other lens must be concave.
• If ω₁ = ω₂, then
• 1/ f₁ + 1/f₂ = 0     ⇒     1/F = 0     ⇒     F = ∞
• Thus combination behaves as a plane glass plate, not as a lens. So the material should be different so that ω₁ ≠ ω₂
• If the combination behaves like a convergent lens, then power of convex lens should be greater than the power of concave lens or f₁ < f₂ hence ω₁ < ω₂ and therefore the convex lens should be of crown and concave lens should be of flint glass.
• The condition which we use here is only for the elimination of longitudinal chromatic aberration, transverse chromatic aberration can not be removed by it.
• If a number of lenses are combined to form achromatic lens, then
• ω₁ / f₁ + ω₂ / f₂ + ω₃ / f₃ + …= 0     or     Σ (ω / f ) = 0

Achromatic combination of two lenses separated by a distance

• If two lenses are separated by a distance d, then the focal length of combination will be

• Since focal length depends on wavelengths