Equipartition theorem

• Let x_i be any coordinate or momenta i.e., x_i = q_i or p_i (i = 1, 2, 3, …)
• If H represents the Hamiltonian, then ensemble average of x_i (∂H/∂x_i)

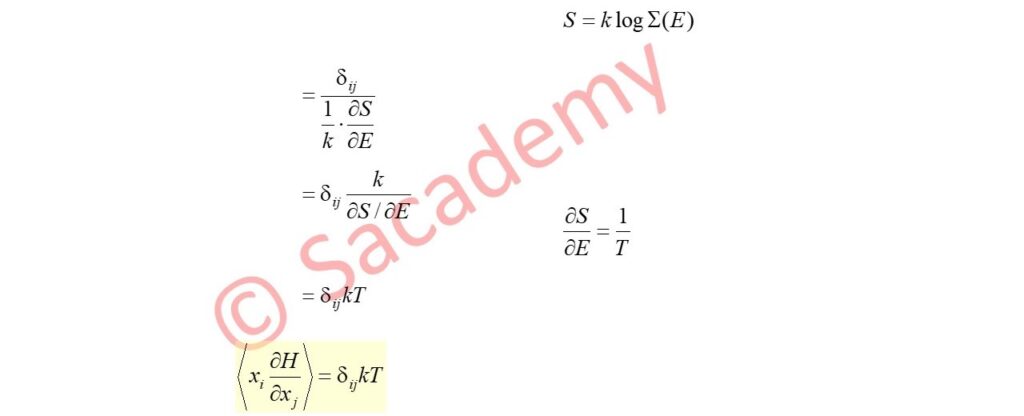
• This is known as generalized equipartition theorem.

Special cases

• If i = j and x_i = p_i, then δ_ij = 1

• If i = j and x_i = q_i, then

• From canonical equation of motion

• Since in classical mechanics the sum of i^th coordinate × i^th component of the geometrical force i.e., Σ q_i ṗ_i is known as the virial
• So above equation is known as Virial theorem.

• In many physical system, Hamiltonian

          H = Σ A_iP_i² + Σ B_iQ_i²

• Here P_i and Q_i are two canonically conjugate variables
• Here A_i and B_i are constants.

Here A_i and B_i are constants.

• Sine P_i and Q_i are canonically conjugate quantities so we can not take them simultaneously.

• If there are f degrees of freedom, then

          < H > = ½ f kT

• Thus each harmonic term in the Hamiltonian contributes ½ kT to the average energy.
• This is known as theorem of equipartition energy.

Statement

• The average energy associated with single variable, coordinate or momentum, which contributes a quadratic term (or square term) to the total energy is ½ kT per molecule in every case.
• Internal energy

          U = < H > = ½ f kT

          C_v = ∂U / ∂T = ½ f k

∴        C_v / k = ½ f

or     C_v / k ∝ f

• It means Heat capacity ∝ Degrees of freedom of the system.