• The entropy of an ideal gas

• Now, we consider two ideal gases with N₁ and N₂ particles respectively.
• These two gases are in two separate container of volumes V₁ and V₂ at the same temperatures i.e., T₁ = T₂ = T and having same density.
• Now, the gases are allowed to mix in a volume V = V₁ + V₂, then the entropy of the composite system (the temperature remain constant) is given by

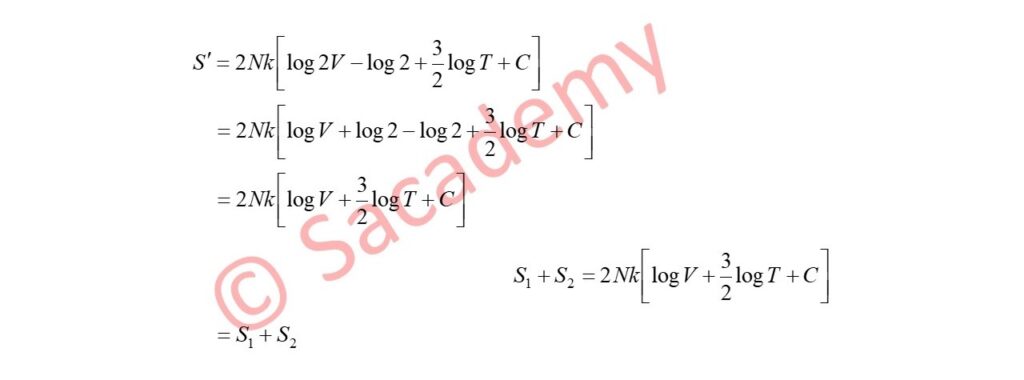
         S = S₁ + S₂

             = N₁k [log V₁ + 3/2 log T + C] + N₂k [log V₂ + 3/2 log T + C]

• If the particles in the two systems are the same and for convenience we take V₁ = V₂ = V, and N₁ = N₂ = N, then total entropy of the system

• This equation shows that by joining two moles of two different gases by removing a partition between them, the entropy of the system increases by an additional factor 2Nk log 2.
• This is called Gibbs paradox.

Explanation

• After the removal of partition, a molecule of each system, being indistinguishable and they can be found anywhere.
• Mixing of two gases within the volume 2V instead of V and hence external parameter become 2V and therefore the possible states of energy are to be calculated with the total volume 2V instead of V.
• This explains the occurrence of the additional factor 2Nklog2.

• But if we take two systems as the same (two molecules of the same gas), then the molecules will be indistinguishable (same as in quantum mechanics).
• In this case we have to correct the formula of entropy by using the idea of indistinguishability to classical approximation.
• If there are n indistinguishable molecules in a system, then the correct formula of entropy is

• Therefore the entropy of the system

• Gibb’s paradox is thus resolved by the concept of quantum mechanics.